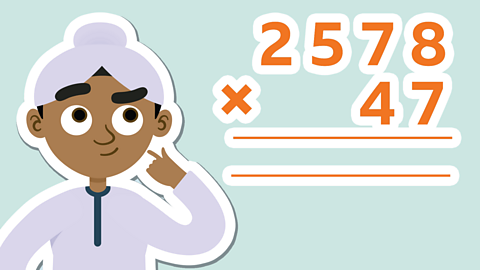
Comparing the size of objects
When you want to describe the size of an object compared to a smaller object, you can use the word ‘times’.
For example, you can say an object is 'five times' as large or 'ten times' as large.
This will depend on how much you have multiplied the original size by.
This blue tower is three times the height of the orange tower.
Quiz: Multiplication as scaling
Test out your knowledge of scaling with this quiz, then read on to complete the page.
Using multiplication for comparing length
Here are some pencils.
There is one yellow pencil and there are three blue pencils.
The blue pencils are all the same length.
You know the length of the blue pencils, but how can you work out the length of the yellow pencil?
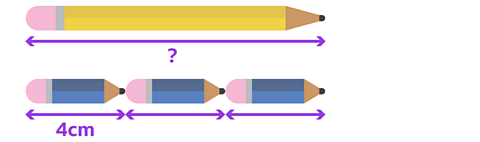
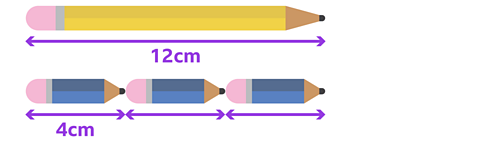
You can work it out using multiplication.
4cm multiplied by 3 is 12cm.
4cm × 3 = 12cm
The yellow pencil is 12cm long. It is three times the length of a blue pencil.
Now look at these pencils.
The yellow pencil is double the length of a green pencil. You could also say it is two times, or twice as long.
How long is the green pencil?
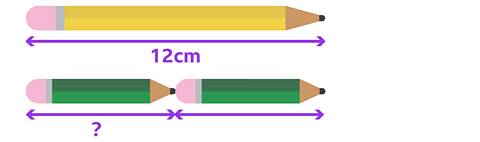
You can work it out using division.
12cm divided by 2 is 6cm.
12cm ÷ 2 = 6cm
The green pencils are 6cm long.
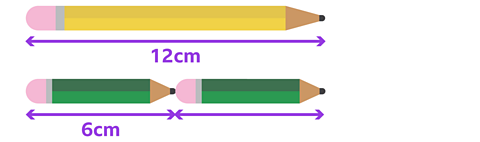
You can write this as a multiplication like this:
2 × 6cm = 12cm
Using multiplication for comparing height
This picture shows how much a plant has grown over time.
When it was planted, it was 10cm tall. How can you work out how tall it is now?
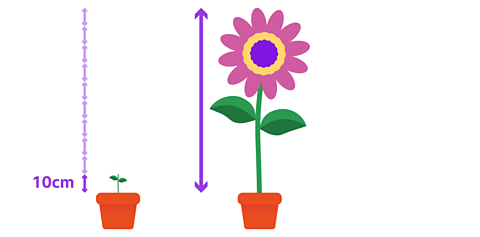
The plant has grown ten times taller. Its original height was 10cm.
10 × 10cm = 100cm
The plant is now 100cm.
Using multiplication to compare time
Sally has taken 5 minutes to eat her lunch. Fred has taken four times as long to eat lunch.
How can you work out how long he took?

You need to multiply 5 minutes by 4.
5 minutes × 4 = 20 minutes
Fred has taken 20 minutes to eat his lunch.
Example 1
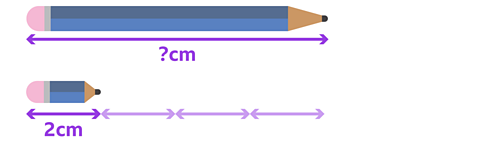
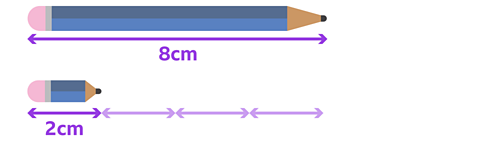
Omi has sharpened her pencil so much that it is now only 2cm long.
Lucas’s pencil is four times as long. How long is Lucas’s pencil?
✓ Lucas's pencil is 8cm long.
His pencil is four times the length of Omi’s.
2cm × 4 = 8 cm
Example 2

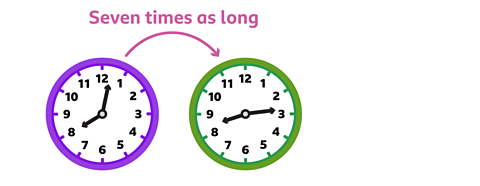
Lucas takes 2 minutes to get to school. It takes Maya 14 minutes to get to school.
How many times longer is Maya's journey to school than Lucas's?
✓ Maya's journey is 7 times longer.
To solve this problem you need to find out how many times you multiply 2 to make 14.
2 × ? = 14
If you know your 2 times tables, you will know:
2 × 7 = 14
Maya takes seven times as long to get to school as Lucas does.
Or, if you remember that division is the inverse of multiplication, you can divide 14 by 2.
14 ÷ 2 = 7
Example 3

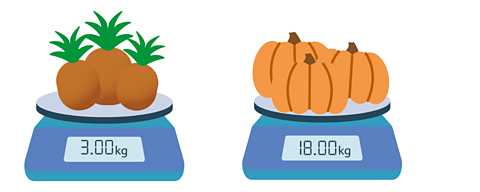
These pineapples have a mass of 3kg and these pumpkins have a mass that is six times the mass of the pineapples.
What is the mass of the pumpkins?
✓ The mass of the pumpkins is 18kg.
You need to multiply 3kg by 6 to find the mass of the pumpkins.
3kg × 6 = 18kg
More on Multiplying and dividing
Find out more by working through a topic
- count24 of 34
- count25 of 34

- count26 of 34

- count27 of 34