Key points
Napier’s method of multiplication is a method used to multiply large numbers when you do not have a calculator.
The advantage of Napier's method is that it only uses multiplication factsThe word 'fact’ is related to the instant recall of knowledge about a number. A multiplication fact for 20 could be 4 x 5. A division fact for 20 could be 20 ÷ 5 = 4 up to 9 x 9 and addition.
You can brush-up on times-tables with this game: Guardians: Defenders of Mathematica.
How to use Napier’s method of long multiplication
Multiplication using Napier's method uses a grid to multiply numbers.
The grid layout depends on the calculation, eg 64 x 77 would need a 2 x 2 grid. For the calculation 321 x 36, either a 3 x 2 grid or a 2 x 3 grid could be used because multiplication is commutativeAn operation is commutative if the order does not matter. Multiplication and addition are commutative, eg 4 × 3 = 3 × 4 and 4 + 3 = 3 + 4 .
A diagonal line is drawn to separate the tens (T) and the units (U) in each box in the grid.
Each cell in the grid represents a two-digit answer to a multiplication productThe result of multiplying one number by another, eg the product of 4 and 5 is 20 since 4 × 5 = 20 made of a tens digit, and a units digit.
Example: calculate 37 x 91

Image caption, Draw a 2 by 2 grid to multiply a 2-digit number by another 2-digit number. Label the grid with figures at the top and the right-hand side.
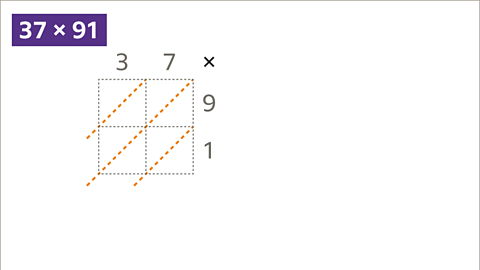
Image caption, Draw the diagonals for each cell in the grid from top right to bottom left and extend outside the grid.
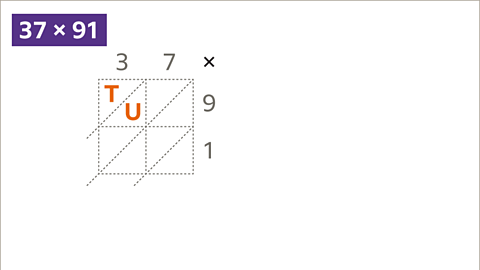
Image caption, Each cell in the grid represents a two-digit answer to a multiplication (product) made of a tens digit, and a units digit.
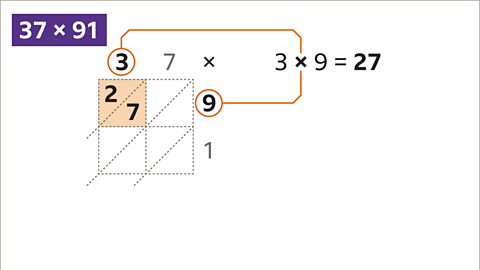
Image caption, This cell holds the product of 3 x 9. 3 x 9 is 27, 2 tens and 7 units.
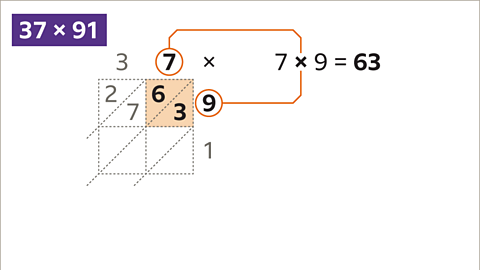
Image caption, This cell holds the product of 7 x 9. 7 x 9 is 63, 6 tens and 3 units.
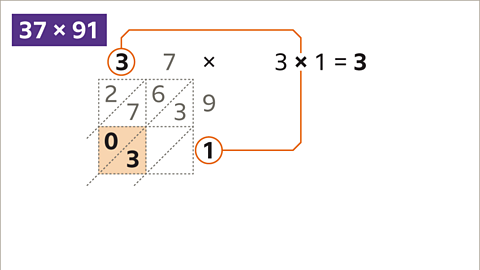
Image caption, This cell holds the product of 3 x 1. 3 x 1 is 3, 0 tens and 3 units.

Image caption, This cell holds the product of 7 x 1. 7 x 1 is 7, 0 tens and 7 units.
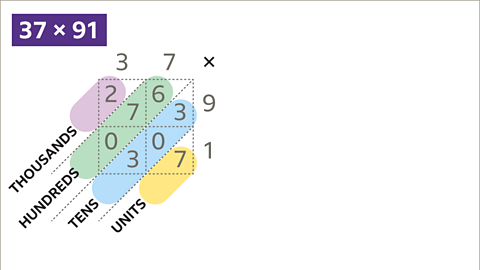
Image caption, The grid is now filled with all the products. The sum of each diagonal gives part of the final answer. From right to left, the first diagonal is the units. The second diagonal is the tens. The third diagonal is the hundreds. The fourth diagonal is the thousands.
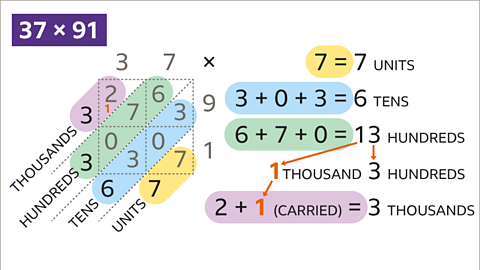
Image caption, Add up each diagonal. Remember to carry when you get a two-digit answer.
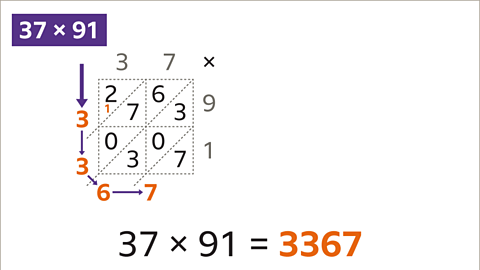
Image caption, Follow the arrows to write the calculation with the answer: 37 x 91 = 3367
1 of 10
Example: calculate 653 x 72
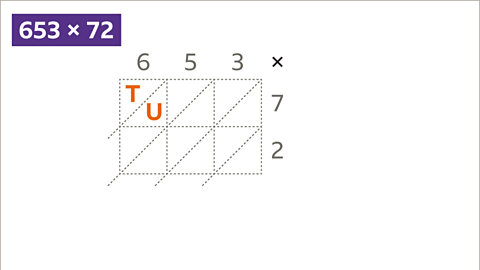
Image caption, Draw and label the grid. Remember each cell in the grid represents a two-digit answer to a multiplication (product) made of a tens digit, and a units digit.
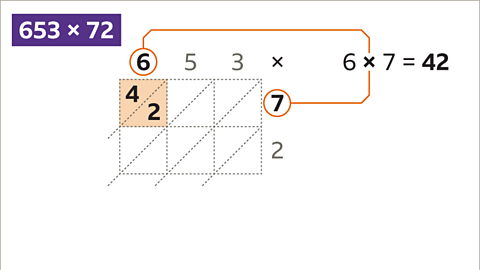
Image caption, The highlighted cell holds the answer to the product 6 x 7. 6 x 7 is 42, 4 tens and 2 units.
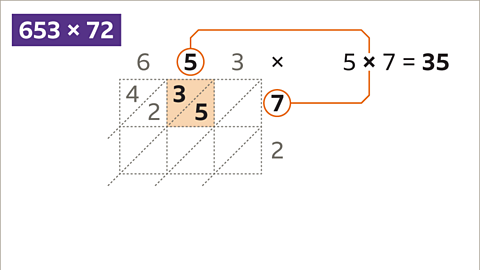
Image caption, This cell holds the answer to the product 5 x 7. 5 x 7 is 35, 3 tens and 5 units.

Image caption, This cell holds the answer to the product 3 x 7. 3 x 7 is 21, 2 tens and 1 unit.
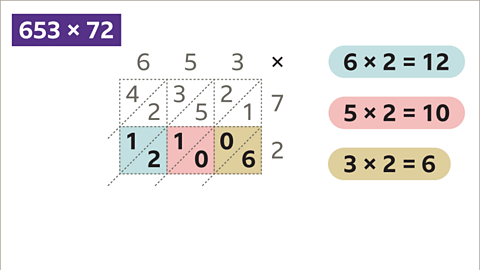
Image caption, The second row of the grid is completed with the three products, 6 x 2, 5 x 2 and 3 x 2.
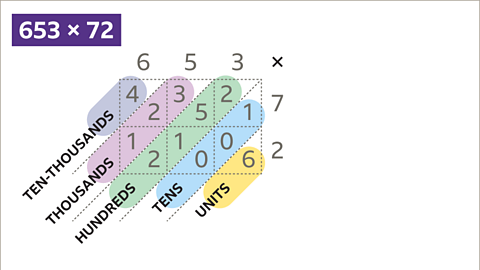
Image caption, The grid is filled with all the products. The sum of each diagonal gives part of the final answer. From right to left, the first diagonal is the units. The second diagonal is the tens. The third diagonal is the hundreds. The fourth diagonal is the thousands. And the fifth diagonal is the ten-thousands.
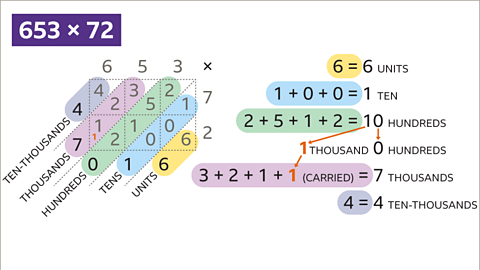
Image caption, Add up each diagonal. Remember to carry when you get a two-digit answer.
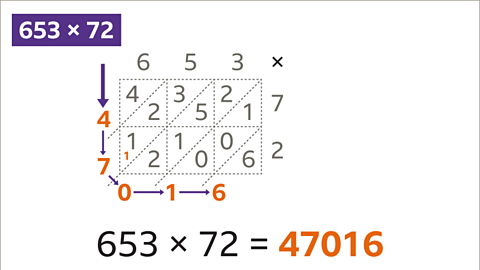
Image caption, Follow the arrows to write the calculation with the answer: 653 x 72 = 47,016
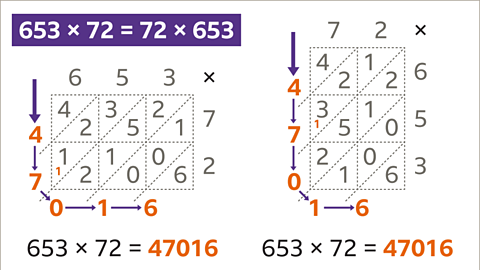
Image caption, Multiplication is commutative so 653 x 72 = 72 x 653. The calculation can be done on a 3 by 2 grid or a 2 by 3 grid.
1 of 9
Practise Napier’s method
Question: spot the mistake
The answer to this calculation is incorrect.Try to find the mistakes in the working. You might need a pen and paper.
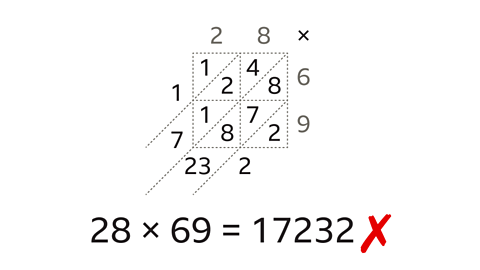
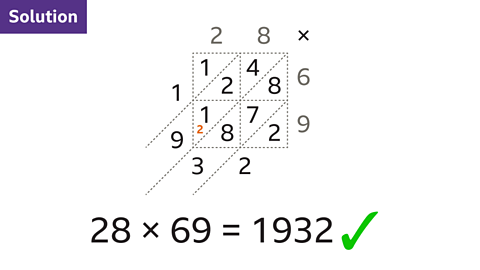
The mistake is that the third diagonal from the right should include a carried digit.
23 is the total of the second diagonal but only the 3 digit should be written there. The 2 is carried to the next diagonal.
7 is the total of the third diagonal (4 + 2 + 1) but there should be a 2 carried and added as well to give a total of 9.
Practise: Napier’s method quiz
Practise long multiplication using Napier's method with this quiz. A pen and paper might be useful to help with your workings out.
Real-world maths

When you do not have a calculator, Napier’s method for long multiplication is one way of calculating your answer.
Multiplication is used to determine areas, quantities for batch production, materials needed for construction projects and much more.
It is a skill used by professionals in catering, engineering, construction and across many businesses. It can be an invaluable skill for the world of work.

Play the Divided Islands game! gamePlay the Divided Islands game!
Using your maths skills, help to build bridges and bring light back to the islands in this free game from BBC Bitesize.

More on Operations - Calculations
Find out more by working through a topic
- count4 of 10
- count5 of 10
- count6 of 10

- count7 of 10